Page 20 - 6637

Chapter 3

Classification of second order linear

PDEs

3.1 Solved Problems

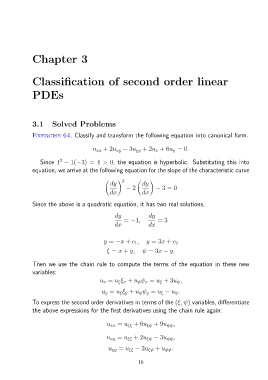
Exercise 64. Classify and transform the following equation into canonical form.

u xx + 2u xy − 3u + 2u + 6u = 0.
yy
x
y
2
Since 1 − 1(−3) = 4 > 0, the equation is hyperbolic. Substituting this into
equation, we arrive at the following equation for the slope of the characteristic curve

2
dy dy
− 2 − 3 = 0
dx dx

Since the above is a quadratic equation, it has two real solutions,

dy dy
= −1, = 3
dx dx

y = −x + c , y = 3x + c 2
1
ξ = x + y, ψ = 3x − y.

Then we use the chain rule to compute the terms of the equation in these new

variables:
u = u ξ + u ψ = u + 3u ,
ψ
ξ x
ψ
ξ
x
x
u = u ξ + u ψ = u − u .
ξ
y
ψ
ξ y
ψ
y
To express the second order derivatives in terms of the (ξ, ψ) variables, differentiate
the above expressions for the first derivatives using the chain rule again:
u xx = u + 6u ξψ + 9u ,
ψψ
ξξ
u xy = u + 2u ξψ − 3u ,
ψψ
ξξ
u yy = u − 2u ξψ + u .
ξξ
ψψ
16