Page 16 - 6637

Supplementary Problems

2 3
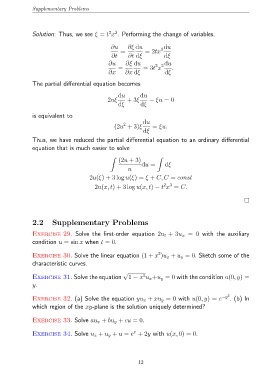
Solution: Thus, we see ξ = t x . Performing the change of variables,

∂u ∂ξ du du
= = 2tx 3
∂t ∂t dξ dξ
∂u ∂ξ du du
2 2
= = 3t x .
∂x ∂x dξ dξ
The partial differential equation becomes

du du
2uξ + 3ξ − ξu = 0
dξ dξ

is equivalent to
du
2
(2u + 3)ξ = ξu.
dξ
Thus, we have reduced the partial differential equation to an ordinary differential
equation that is much easier to solve

(2u + 3)
Z Z
du = dξ
u
2u(ξ) + 3 log u(ξ) = ξ + C, C = const

2 3
2u(x, t) + 3 log u(x, t) − t x = C.

2.2 Supplementary Problems

Exercise 29. Solve the first-order equation 2u + 3u = 0 with the auxiliary
t
x
condition u = sin x when t = 0.

2
Exercise 30. Solve the linear equation (1 + x )u + u = 0. Sketch some of the
x
y
characteristic curves.
√
2
Exercise 31. Solve the equation 1 − x u +u = 0 with the condition u(0, y) =
y
x
y.
Exercise 32. (a) Solve the equation yu + xu = 0 with u(0, y) = e −y 2 . (b) In
y
x
which region of the xy-plane is the solution uniquely determined?

Exercise 33. Solve au + bu + cu = 0.
x
y
x
Exercise 34. Solve u + u + u = e + 2y with u(x, 0) = 0.
y
x

12