Page 97 - 4811

Application of Laplace Transform
.

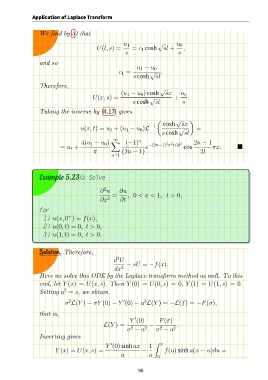
We find by 3) that
u 1 √ u 0
U(l, s) = = c cosh sl + ,
1
s s
and so
u − u 0
1
c = √ .
1
s cosh sl
Therefore,
√
(u − u ) cosh sx u
U(x, s) = 1 0 √ + 0 .
s cosh sl s
Taking the inverse by (4.17) gives

( √ )
cosh sx
−1
u(x, t) = u + (u − u )L √ =
1
0
0
s cosh sl
∞
4(u − u ) ∑ (−1) n 2 2 2 2n − 1
1
0
= u + e −(2n−1) π t/4l cos πx.
1
π (2n − 1) 2l
n=1
. . . .
.
Example 5.23, Solve
2
∂ u ∂u
= , 0 < x < 1, t > 0,
∂x 2 ∂t
for

+
1) u(x, 0 ) = f(x),
2) u(0, t) = 0, t > 0,
3) u(1, t) = 0, t > 0.
.
. . . . .
Solution. Therefore,
2
d U
− sU = −f(x).
dx 2
Here we solve this ODE by the Laplace transform method as well. To this
end, let Y (x) = U(x, s). Then Y (0) = U(0, s) = 0, Y (1) = U(1, s) = 0.
2
Setting a = s, we obtain
2
2
′
σ L(Y ) − σY (0) − Y (0) − a L(Y ) = −L(f) = −F(σ),
that is,
Y (0) F(σ)
′
L(Y ) = − .
2
2
σ − a 2 σ − a 2
Inverting gives
∫ x
′
Y (0) sinh ax 1
Y (x) = U(x, s) = − f(u) sinh a(x − u)du =
. . . . a a 0

96