Page 100 - 4811

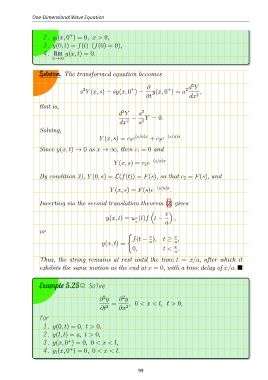
One-Dimensional Wave Equation
.

+
2. y (x, 0 ) = 0, x > 0,
t
3. y(0, t) = f(t) (f(0) = 0),
4. lim y(x, t) = 0.
x→∞
. . . . .
.
Solution. The transformed equation becomes

2
∂ d Y
2 + + 2
s Y (x, s) − sy(x, 0 ) − y(x, 0 ) = a ,
∂t dx 2
that is,
2
d Y s 2
− Y = 0.
dx 2 a 2
Solving,

Y (x, s) = c e (s/a)x + c e −(s/a)x .
1
2
Since y(x, t) → 0 as x → ∞, then c = 0 and
1
Y (x, s) = c e −(s/a)x .
2

By condition 3), Y (0, s) = L(f(t)) = F(s), so that c = F(s), and
2

Y (x, s) = F(s)e −(s/a)x .

Inverting via the second translation theorem (2) gives
( )
x
y(x, t) = u x (t)f t − ,
a a
or
{
x
x
f(t − ), t ≥ ,
y(x, t) = a a
x
0, t < .
a
Thus, the string remains at rest until the time t = x/a, after which it
. . . . exhibits the same motion as the end at x = 0, with a time delay of x/a.
.

Example 5.25, Solve

2
2
∂ y ∂ y
= , 0 < x < l, t > 0,
∂t 2 ∂x 2
for

1. y(0, t) = 0, t > 0,
2. y(l, t) = a, t > 0,
+
3. y(x, 0 ) = 0, 0 < x < l,
+
4. y (x, 0 ) = 0, 0 < x < l.
t
. . . . .

99