Page 60 - 4811

Fourier-Mellin formula

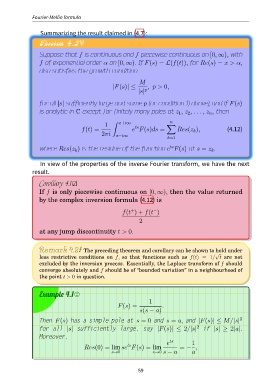
Summarizing the result claimed in (4.7):

Theorem 4.2È

Suppose that f is continuous and f piecewise continuous on [0, ∞), with

f of exponential order α on [0, ∞). If F(s) = L(f(t)), for Re(s) = x > α,
also satisfies the growth condition

M
|F(s)| ≤ , p > 0,
|s| p

for all |s| sufficiently large and some p (or condition 1) above), and if F(s)
is analytic in C except for finitely many poles at z , z , . . . , z , then
n
2
1
∫ n
1 x+i∞ ∑
ts
f(t) = e F(s)ds = Res(z ), (4.12)
k
2πi
x−i∞
k=1
ts
where Res(z ) is the residue of the function e F(s) at s = z .
k
k
. . . . . . .
In view of the properties of the inverse Fourier transform, we have the next
result.

Corollary 4.12
If f is only piecewise continuous on [0, ∞), then the value returned
by the complex inversion formula (4.12) is

+
f(t ) + f(t )
−
2

at any jump discontinuity t > 0.
. . . . .

Remark 4.2E The preceding theorem and corollary can be shown to hold under
√
less restrictive conditions on f, so that functions such as f(t) = 1/ t are not
excluded by the inversion process. Essentially, the Laplace transform of f should
converge absolutely and f should be of “bounded variation” in a neighbourhood of

. . . . . . the point t > 0 in question.

Example 4.1,
1
F(s) = .
s(s − a)

2
Then F(s) has a simple pole at s = 0 and s = a, and |F(s)| ≤ M/|s|
2
for all |s| sufficiently large, say |F(s)| ≤ 2/|s| if |s| ≥ 2|a|.
Moreover,
e ts 1
ts
Res(0) = lim se F(s) = lim = − ,
s→0 s→0 s − a a
. . . . .

59