Page 56 - 4811

Fourier-Mellin formula

In order to calculate the integral in (4.3) and so determine the inverse of the

Laplace transform F(s), we employ the standard methods of contour
integration.
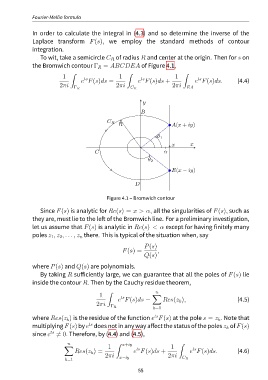
To wit, take a semicircle C of radius R and center at the origin. Then for s on
R
the Bromwich contour Γ = ABCDEA of Figure 4.1,
R
∫ ∫ ∫
1 1 1
ts
ts
ts
e F(s)ds = e F(s)ds + e F(s)ds. (4.4)
2πi 2πi 2πi
Γ R C R EA
y
B

C R
R A(x + iy)

θ 1
x x
C α
θ 2
E(x − iy)

D
B C D
Figure 4.1 – Bromwich contour

Since F(s) is analytic for Re(s) = x > α, all the singularities of F(s), such as
they are, must lie to the left of the Bromwich line. For a preliminary investigation,
let us assume that F(s) is analytic in Re(s) < α except for having finitely many
poles z , z , . . . , z there. This is typical of the situation when, say
2
1
n
P(s)
F(s) = ,
Q(s)

where P(s) and Q(s) are polynomials.
By taking R sufficiently large, we can guarantee that all the poles of F(s) lie

inside the contour R. Then by the Cauchy residue theorem,

∫ n
1 ∑
ts
e F(s)ds = Res(z ), (4.5)
k
2πi
Γ R
k=1
ts
where Res(z ) is the residue of the function e F(s) at the pole s = z . Note that
k
k
ts
multiplying F(s) by e does not in any way affect the status of the poles z of F(s)
k
ts
since e ̸= 0. Therefore, by (4.4) and (4.5),
n ∫ x+iy ∫
∑ 1 1
ts
ts
Res(z ) = e F(s)ds + e F(s)ds. (4.6)
k
2πi 2πi
k=1 x−iy C R
55