Page 26 - 4749

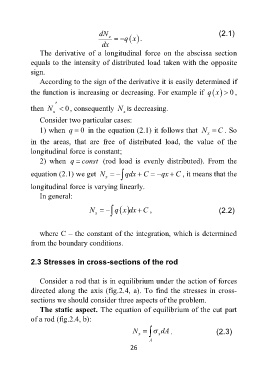
dN (2.1)
x   q   x .
dx
The derivative of a longitudinal force on the abscissa section
equals to the intensity of distributed load taken with the opposite
sign.
According to the sign of the derivative it is easily determined if

the function is increasing or decreasing. For example if   0q x  ,

then N  0, consequently N is decreasing.
x x
Consider two particular cases:
0
1) when q  in the equation (2.1) it follows that N  C . So
x
in the areas, that are free of distributed load, the value of the
longitudinal force is constant;
2) when q  const (rod load is evenly distributed). From the

equation (2.1) we get N   qdx  C  qx  C , it means that the

x
longitudinal force is varying linearly.
In general:
N    q   x dx C , (2.2)
x

where C – the constant of the integration, which is determined
from the boundary conditions.

2.3 Stresses in cross-sections of the rod

Consider a rod that is in equilibrium under the action of forces
directed along the axis (fig.2.4, a). To find the stresses in cross-
sections we should consider three aspects of the problem.
The static aspect. The equation of equilibrium of the cut part
of a rod (fig.2.4, b):
x 
N   x dA . (2.3)
A
26