Page 25 - 4749

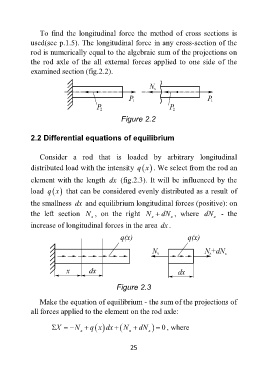
To find the longitudinal force the method of cross sections is
used(see p.1.5). The longitudinal force in any cross-section of the
rod is numerically equal to the algebraic sum of the projections on
the rod axle of the all external forces applied to one side of the
examined section (fig.2.2).

Figure 2.2

2.2 Differential equations of equilibrium

Consider a rod that is loaded by arbitrary longitudinal
q
distributed load with the intensity   x . We select from the rod an
element with the length dx (fig.2.3). It will be influenced by the
load   x that can be considered evenly distributed as a result of
q
the smallness dx and equilibrium longitudinal forces (positive): on
the left section N , on the right N  dN , where dN - the
x x x x
increase of longitudinal forces in the area dx .

Figure 2.3
Make the equation of equilibrium - the sum of the projections of
all forces applied to the element on the rod axle:

 X   N  q   x dx   N  dN  0 , where
x x x

25