Page 58 - 4560

The system (5.15) will have a nonzero solution if its determinant
is zero:
0 1 0 1

k 0 1 0
 0 .
sin kl coskl l 1
sin kl coskl 0 0
Expanding this determinant, we obtain a transcendental equation
for determining k :

tgkl  kl . (5.16)
The smallest root of this equation defines the first critical force.
We have kl  4,493 1,43 , then

2
4,493 EJ  2 EJ
F  min  min ,
кр 2 2
l 0,7l 

namely   0,699 0,7 . The coefficients for other ways of
fixing the rod load and get the same way, considering the
rigorous formulation of the boundary value problem of
longitudinal bending for each case.
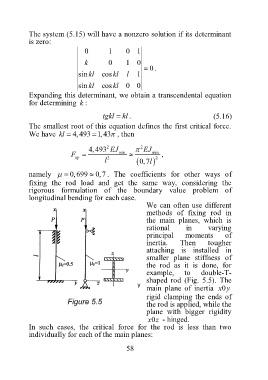
We can often use different
methods of fixing rod in
the main planes, which is
rational in varying
principal moments of
inertia. Then tougher
attaching is installed in
smaller plane stiffness of
the rod as it is done, for
example, to double-T-
shaped rod (Fig. 5.5). The
у
main plane of inertia 0x y
rigid clamping the ends of
Figure 5.5 the rod is applied, while the
plane with bigger rigidity

0 x z - hinged.
In such cases, the critical force for the rod is less than two
individually for each of the main planes:

58