Page 56 - 4560

where A – most uncertain bent axis deviation from a straight line
status.
On the length of the rod between the hinge supports half-wave
sinusoid fit as deviations will not be at x  and x  .
l
0
If n more than one, then bent rod axis, described by the equation
n x
y  A sin , (5.10)
l
contains more than one half-wave sine wave-number n (rys.5.3).
Under these conditions require a greater force is critical to keep
the rod bent in a state of n half-waves, as seen from equation
(5.7).
So, if the gripping force P  P , the rod has only one (straight)
кр
form of equilibrium that is stable.
If P  P , in addition, there is another with a straight (curved)
кр
shape equilibrium and rectilinear form of equilibrium is unstable
and stable equilibrium shape is distorted.
It should be noted that the critical force does not depend on the
characteristics of the material of the rod.

5.3 Influence of rod fixing on the value of the critical
force

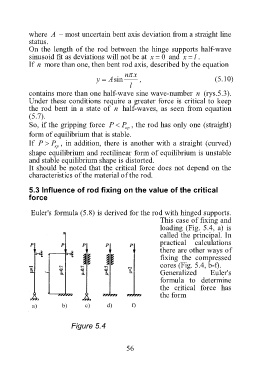
Euler's formula (5.8) is derived for the rod with hinged supports.
This case of fixing and
loading (Fig. 5.4, a) is
called the principal. In
practical calculations
there are other ways of
fixing the compressed
cores (Fig. 5.4, b-f).
Generalized Euler's
formula to determine
the critical force has
the form

Figure 5.4

56