Page 54 - 4560

For steel rods k is taken from 1.8 to 3; rod iron k  5 5,5 ,
ст ст
for wood rods k  3 3,2 .
ст
To prevent the loss of stability of structures, it is necessary to
enforce stability conditions (5.1), and it needs to be able to
determine the critical load.

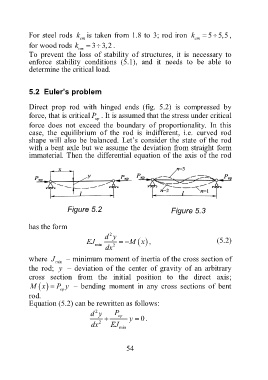
5.2 Euler’s problem

Direct prop rod with hinged ends (fig. 5.2) is compressed by
force, that is critical P . It is assumed that the stress under critical
кр
force does not exceed the boundary of proportionality. In this
case, the equilibrium of the rod is indifferent, i.e. curved rod
shape will also be balanced. Let’s consider the state of the rod
with a bent axle but we assume the deviation from straight form
immaterial. Then the differential equation of the axis of the rod

Figure 5.2 Figure 5.3

has the form
2
d y
EJ   M   x , (5.2)
min 2
dx

where J – minimum moment of inertia of the cross section of
min
the rod; y – deviation of the center of gravity of an arbitrary
cross section from the initial position to the direct axis;
M   x  P y – bending moment in any cross sections of bent
кр
rod.
Equation (5.2) can be rewritten as follows:
2
d y P кр
 y  0.
dx 2 EJ
min

54