Page 59 - 4549

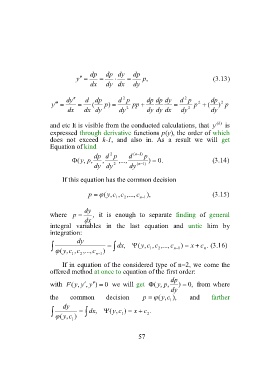
dp dp dy dp
 
y     , p (3.13)
dx dy dx dy

y d  d dp d 2 p dp dp dy d 2 p dp
2
  
y   ( p)  pp   p  ( ) 2 p
dx dx dy dy 2 dy dy dx dy 2 dy

and etc It is visible from the conducted calculations, that y (k ) is
expressed through derivative functions р(у), the order of which
does not exceed k-1, and also in. As a result we will get
Equation of kind
dp d 2 p d (n ) 1  p
( y , , p , ,..., )  . 0 (3.14)
dy dy 2 dy (n ) 1 

If this equation has the common decision


p  (y ,c ,c ,...,c ), (3.15)
1 2 n 1 

dy
where p  , it is enough to separate finding of general
dx
integral variables in the last equation and untie him by
integration:
dy
  dx ,  ,( cy 1 ,c 2 ,...,c n 1 )  x  c n . (3.16)

 (y ,c ,c ,...,c )
1 2 n 1

If in equation of the considered type of n=2, we come the
offered method at once to equation of the first order:
dp
with F (y ,  y ,  y )  0 we will get  y , , p )  , 0 from where
(
dy
the common decision p  (y ,c ), and farther
1
dy
  dx ,  ,( cy 1 )  x  c 2 .

 (y ,c )
1

57