Page 37 - 4549

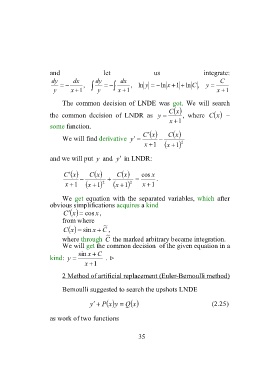
and let us integrate:
dy dx dy dx C
  ,     , ln y   ln x 1  ln C , y 
y x  1 y x  1 x  1

The common decision of LNDE was got. We will search
C  x
the common decision of LNDR as y  , where   xC –
x  1
some function.
C   x C  x
We will find derivative  y  
x  1  x  1 2

and we will put y and y in LNDR:

C   x C  x C  x cos x
   .
x  1  x  1 2  x  1 2 x  1

We get equation with the separated variables, which after
obvious simplifications acquires a kind
C   x  cos x ,
from where
~
C  x  sin x  C ,
~
where through C the marked arbitrary became integration.
We will get the common decision of the given equation in a
sin  Cx
kind: y  . 
x  1

2 Method of artificial replacement (Euler-Bernoulli method)

Bernoulli suggested to search the upshots LNDE

y   P  yx  Q   x (2.25)

as work of two functions

35