Page 33 - 4549

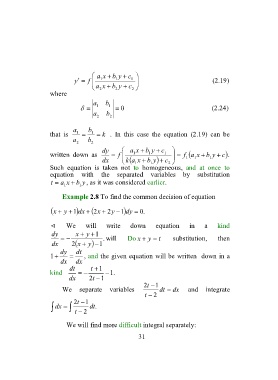
 a x  b y  c 
 y   f  1 1 1  (2.19)

 a 2 x  b 2 y  c 2 
where
a 1 b 1
   0 (2.24)
a b
2 2

a b
that is 1  1  k . In this case the equation (2.19) can be
a b
2 2
dy  a x  b y  c 
written down as  f  1 1 1   f  xa  b y   c .
dx  k  xa 1  b 1 y  c 2  1 1 1


Such equation is taken not to homogeneous, and at once to
equation with the separated variables by substitution
t  a x  b y , as it was considered earlier.
1 1

Example 2.8 To find the common decision of equation

  yx   1 dx  2 x 2 y  1 dy  . 0

 We will write down equation in a kind
dy x  y  1
  . will Do x  y  t substitution, then
dx  2 x  y  1
dy dt
1   , and the given equation will be written down in a
dx dx
dt t  1
kind    . 1
dx 2 t 1
2 t 1
We separate variables dt  dx and integrate
t  2
2t 1
 dx   dt .
t  2

We will find more difficult integral separately:
31