Page 29 - 4549

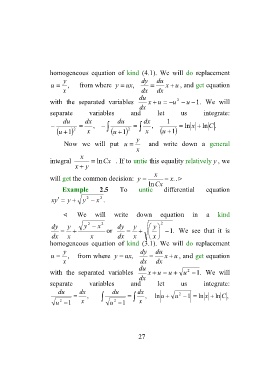
homogeneous equation of kind (4.1). We will do replacement
y dy du
u  , from where y  ux,  x  u , and get equation
x dx dx
du
with the separated variables x  u  u 2  u  1. We will
dx
separate variables and let us integrate:
du dx du dx 1
  ,     ,  ln x  lnC .
 1u  2 x  1u  2 x  1u 
y
Now we will put u  and write down a general
x
x
integral  ln Cx . If to untie this equality relatively y , we
x  y
x
will get the common decision: y   . x .
lnCx
Example 2.5 To untie differential equation
2
y x  y  y  x 2 .


 We will write down equation in a kind
2
dy y y  x 2 dy y  y  2
  or      . 1 We see that it is
dx x x dx x  x 
homogeneous equation of kind (3.1). We will do replacement
y dy du
u  , from where y  ux,  x  u , and get equation
x dx dx
du
with the separated variables x  u  u  u 2  . 1 We will
dx
separate variables and let us integrate:
du dx du dx 2
,
 ,    , ln u  u 1  ln x  ln C
u 2 1 x u 2 1 x

27