Page 80 - 4498

them, while the positions of the
atoms inside the unit cell are
described by the set of atomic
positions measured from a lattice

point.
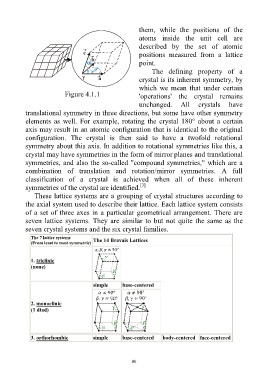
The defining property of a
crystal is its inherent symmetry, by

which we mean that under certain
Figure 4.1.1 'operations' the crystal remains

unchanged. All crystals have
translational symmetry in three directions, but some have other symmetry

elements as well. For example, rotating the crystal 180° about a certain
axis may result in an atomic configuration that is identical to the original
configuration. The crystal is then said to have a twofold rotational

symmetry about this axis. In addition to rotational symmetries like this, a
crystal may have symmetries in the form of mirror planes and translational
symmetries, and also the so-called "compound symmetries," which are a

combination of translation and rotation/mirror symmetries. A full
classification of a crystal is achieved when all of these inherent
[3]
symmetries of the crystal are identified.
These lattice systems are a grouping of crystal structures according to
the axial system used to describe their lattice. Each lattice system consists
of a set of three axes in a particular geometrical arrangement. There are
seven lattice systems. They are similar to but not quite the same as the

seven crystal systems and the six crystal families.
The 7 lattice systems The 14 Bravais Lattices
(From least to most symmetric)

1. triclinic

(none)

simple base-centered

2. monoclinic
(1 diad)

3. orthorhombic simple base-centered body-centered face-centered

80