Page 80 - 4263

Surfaces of the second order

z

. . . . . . . b y
a 0

x

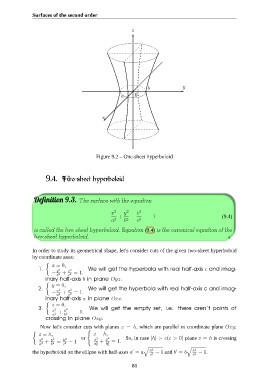
Figure 9.2 – One-sheet hyperboloid

9.4. Two-sheet hyperboloid

Definition 9.3. The surface with the equation

x 2 y 2 z 2
+ − = −1 (9.4)
a 2 b 2 c 2

is called the two-sheet hyperboloid. Equation (9.4) is the canonical equation of the
two-sheet hyperboloid. ✓

In order to study its geometrical shape, let’s consider cuts of the given two-sheet hyperboloid
by coordinate axes:
{
x = 0,
1. y 2 2 We will get the hyperbola with real half-axis c and imag-
− 2 + z 2 = 1.
b c
inary half-axis b in plane Oyz.
{
y = 0,
2. x 2 z 2 We will get the hyperbola with real half-axis c and imag-
− 2 + 2 = 1.
a c
inary half-axis a in plane Oxz.
{
z = 0,
3. We will get the empty set, i.e. there aren’t points of
x 2 y 2
a 2 + b 2 = −1.
crossing in plane Oxy.
Now let’s consider cuts with planes z = h, which are parallel to coordinate plane Oxy:
{
{
z = h, z = h,
2
x 2 2 + y 2 2 = h 2 − 1 or x 2 2 + y 2 2 = 1. So, in case |h| > c(c > 0) plane z = h is crossing
a b c a 0 b 0
√ √
2
2
the hyperboloid on the ellipse with half-axes a = a h 2 − 1 and b = b h 2 − 1.
′
′
c c
80