Page 82 - 4263

Surfaces of the second order

z

y
. . . . .
0
x

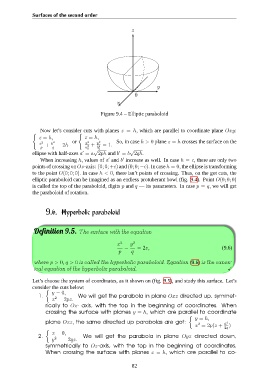
Figure 9.4 – Elliptic paraboloid

Now let’s consider cuts with planes z = h, which are parallel to coordinate plane Oxy:
{ {
z = h, z = h,
2
x 2 + y 2 = 2h or x 2 2 + y 2 = 1. So, in case h > 0 plane z = h crosses the surface on the
p q a b
0
√ 0 √
′
ellipse with half-axes a = a 2ph and b = b 2qh.
′
′
When increasing h, values of a and b increase as well. In case h = c, there are only two
′
points of crossing on Oz-axis: (0; 0; +c) and (0; 0; −c). In case h = 0, the ellipse is transforming
to the point O(0; 0; 0). In case h < 0, there isn’t points of crossing. Thus, on the got cuts, the
elliptic paraboloid can be imagined as an endless protuberant bowl (fig. 9.4). Point O(0; 0; 0)
is called the top of the paraboloid, digits p and q — its parameters. In case p = q, we will get
the paraboloid of rotation.

9.6. Hyperbolic paraboloid

Definition 9.5. The surface with the equation

x 2 y 2
− = 2z, (9.6)
p q

where p > 0, q > 0 is called the hyperbolic paraboloid. Equation (9.6) is the canon-
ical equation of the hyperbolic paraboloid. ✓

Let’s choose the system of coordinates, as it shown on (fig. 9.5), and study this surface. Let’s
consider the cuts below:
{
y = 0,
1. We will get the parabola in plane Oxz directed up, symmet-
2
x = 2pz.
rically to Oz- axis, with the top in the beginning of coordinates. When
crossing the surface with planes y = h, which are parallel to coordinate
{
y = h,
plane Oxz, the same directed up parabolas are got: 2
2
x = 2p(z + h )
2q
{
x = 0,
2. We will get the parabola in plane Oyz directed down,
2
y = −2qz.
symmetrically to Oz-axis, with the top in the beginning of coordinates.
When crossing the surface with planes x = h, which are parallel to co-
82