Page 84 - 4263

Surfaces of the second order

z

y
. . . . .
0
x

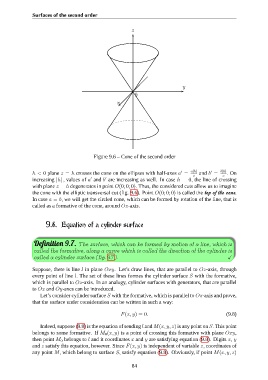
Figure 9.6 – Cone of the second order

′
h < 0 plane z = h crosses the cone on the ellipses with half-axes a = a|h| and b = b|h| . On
′
c c
increasing |h| , values of a and b are increasing as well. In case h = 0, the line of crossing
′
′
with plane z = h degenerates in point O(0; 0; 0). Thus, the considered cuts allow us to imagine
the cone with the elliptic transversal cut (fig. 9.6). Point O(0; 0; 0) is called the top of the cone.
In case a = b, we will get the circled cone, which can be formed by rotation of the line, that is
called as a formative of the cone, around Oz-axis.

9.8. Equation of a cylinder surface

Definition 9.7. The surface, which can be formed by motion of a line, which is
called the formative, along a curve which is called the direction of the cylinder is
called a cylinder surface (fig. 9.7). ✓

Suppose, there is line l in plane Oxy. Let’s draw lines, that are parallel to Oz-axis, through
every point of line l. The set of these lines formes the cylinder surface S with the formative,
which is parallel to Oz-axis. In an analogy, cylinder surfaces with generators, that are parallel
to Ox and Oy-axes can be introduced.
Let’s consider cylinder surface S with the formative, which is parallel to Oz-axis and prove,
that the surface under consideration can be written in such a way:

F(x, y) = 0. (9.8)

Indeed, suppose (9.8) is the equation of sending l and M(x, y, z) is any point on S. This point
belongs to some formative. If M 0 (x, y) is a point of crossing this formative with plane Oxy,
then point M 0 belongs to l and it coordinates x and y are satisfying equation (9.8). Digits x, y
and z satisfy this equation, however. Since F(x, y) is independent of variable z, coordinates of
any point M, which belong to surface S, satisfy equation (9.8). Obviously, if point M(x, y, z)

84