Page 81 - 4263

Elliptic paraboloid

z

c
y
. . . . . . .
0
−c

x

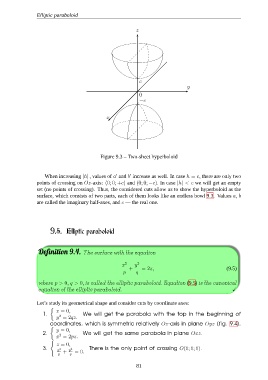
Figure 9.3 – Two-sheet hyperboloid

When increasing |h| , values of a and b increase as well. In case h = c, there are only two
′
′
points of crossing on Oz-axis: (0; 0; +c) and (0; 0; −c). In case |h| < c we will get an empty
set (no points of crossing). Thus, the considered cuts allow us to show the hyperboloid as the
surface, which consists of two parts, each of them looks like an endless bowl 9.3. Values a, b
are called the imaginary half-axes, and c — the real one.

9.5. Elliptic paraboloid

Definition 9.4. The surface with the equation

x 2 y 2
+ = 2z, (9.5)
p q
where p > 0, q > 0, is called the elliptic paraboloid. Equation (9.5) is the canonical
equation of the elliptic paraboloid. ✓

Let’s study its geometrical shape and consider cuts by coordinate axes:
{
x = 0,
1. We will get the parabola with the top in the beginning of
2
y = 2qz.
coordinates, which is symmetric relatively Oz-axis in plane Oyz (fig. 9.4).
{
y = 0,
2. We will get the same parabola in plane Oxz.
2
x = 2pz.
{
z = 0,
3. x 2 y 2 There is the only point of crossing O(0; 0; 0).
p + q = 0.
81