Page 75 - 4263

Angle between two lines in space

is a formula for calculating of distance from a point to a line in space.

8.8. Angle between two lines in space

Let’s consider two lines (l 1 ) and (l 2 ), that are given with the corresponding equations: x−x 1 =
m 1
= and = = . Regardless of any location of lines (l 1 ) and (l 2 ) in space,
y−y 1 z−z 1 x−x 2 y−y 2 z−z 2
n 1 p 1 m 2 n 2 p 2
−→
one out of two angles between them equals to angle φ between their directed vectors s 1 =
−→
(m 1 , n 1 , p 1 ) and s 2 = (m 2 , n 2 , p 2 ), and can be calculated following the formula: cos φ =
−→ −→
s 1 · s 2 or in the view of coordinates:
−→ −→
| s 1|·| s 2|
m 1 m 2 + n 1 n 2 + p 1 p 2
cos φ = √ √ (8.22)
2 2 2 2 2 2
m + n + p · m + n + p
1 1 1 2 2 2
is a formula for calculating of cosine angle between lines in space. Let’s consider the condi-
tions of parallelity and perpendicularity of two lines. Lines (l 1 ) and (l 2 ) are parallel when and
−→
− →
only when their directed vectors s 1 = (m 1 , n 1 , p 1 ) and s 2 = (m 2 , n 2 , p 2 ) are colinear. The
following condition of parallelity of two lines can be expressed:

m 1 n 1 p 1
= = . (8.23)
m 2 n 2 p 2
−→
Lines (l 1 ) and (l 2 ) are perpendicular when and only when their directed vectors s 1 =
(m 1 , n 1 , p 1 ) and s 2 = (m 2 , n 2 , p 2 ) are perpendicular. The following condition of perpendicu-
−→
larity of two lines can be expressed:

m 1 m 2 + n 1 n 2 + p 1 p 2 = 0. (8.24)

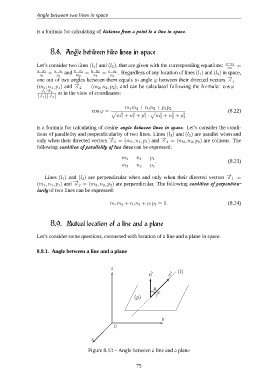
8.9. Mutual location of a line and a plane

Let’s consider some questions, connected with location of a line and a plane in space.

8.9.1. Angle between a line and a plane

z
−→ −→ (l)
n s

θ
φ
(p)

y
. . . . . . . . . . .
0

x

Figure 8.13 – Angle between a line and a plane

75