Page 85 - 4263

Equation of a cylinder surface

z

M
S

y
. . . . . . . . . M 0
0
(l)

x

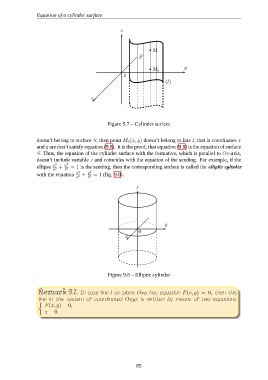
Figure 9.7 – Cylinder surface

doesn’t belong to surface S, then point M 0 (x, y) doesn’t belong to line l, that is coordinates x
and y are don’t satisfy equation (9.8). It is the proof, that equation (9.8) is the equation of surface
S. Thus, the equation of the cylinder surface with the formative, which is parallel to Oz-axis,
doesn’t include variable z and coincides with the equation of the sending. For example, if the
ellipse x 2 2 + y 2 2 = 1 is the sending, then the corresponding surface is called the elliptic cylinder
a b
with the equation x 2 2 + y 2 2 = 1 (fig. 9.8).
a b
z

y
. . . . .
0
x

Figure 9.8 – Elliptic cylinder

Remark 9.1. In case line l on plane Oxy has equation F(x, y) = 0, then this
line in the system of coordinated Oxyz is written by means of two equations:
{
F(x, y) = 0,

z = 0.

85