Page 68 - 4263

Analytic geometry in space

abz + acy = 0 or bcx + acy + abz = abc. Having divided into abc, we will get the equation:

x y z
+ + = 1, (8.6)
a b c
which is called the equation of the plane in segments.

z

3

(p)
y
−4 . . . . . . . . .
0

6
x

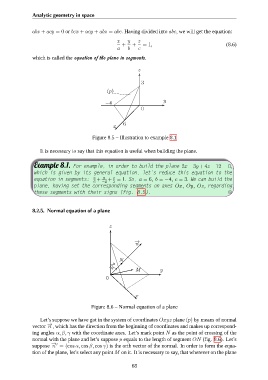
Figure 8.5 – Illustration to example 8.1

It is necessary to say that this equation is useful when building the plane.

Example 8.1. For example, in order to build the plane 2x − 3y + 4z − 12 = 0,
which is given by its general equation, let’s reduce this equation to the
z
equation in segments: x + y + = 1. So, a = 6, b = −4, c = 3. We can build the
6 −4 3
plane, having set the corresponding segments on axes Ox, Oy, Oz, regarding
these segments with their signs (fig. 8.5). ,

8.2.5. Normal equation of a plane

z

− →
n

N
− 0 n
→
. . . . . . . . . M y
0

x

Figure 8.6 – Normal equation of a plane

Let’s suppose we have got in the system of coordinates Oxyz plane (p) by means of normal
−→
vector n , which has the direction from the beginning of coordinates and makes up correspond-
ing angles α, β, γ with the coordinate axes. Let’s mark point N as the point of crossing of the
normal with the plane and let’s suppose p equals to the length of segment ON (fig. 8.6). Let’s
suppose n = (cos α, cos β, cos γ) is the orth vector of the normal. In order to form the equa-
−→′
tion of the plane, let’s select any point M on it. It is necessary to say, that wherever on the plane

68