Page 78 - 4234

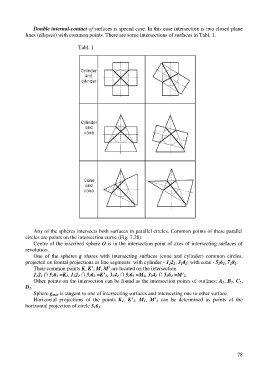
Double internal-contact of surfaces is special case. In this case intersection is two closed plane
lines (ellipses) with common points. There are some intersections of surfaces in Tabl. 1.

Tabl. 1

Any of the spheres intersects both surfaces in parallel circles. Common points of these parallel
circles are points on the intersection curve (Fig. 7.28).
Centre of the inscribed sphere O is in the intersection point of axes of intersecting surfaces of
revolution.
One of the spheres g shares with intersecting surfaces (cone and cylinder) common circles,
projected on frontal projections as line segments: with cylinder - 1 22 2, 3 24 2; with cone - 5 26 2, 7 28 2.
Their common points K, K’, M, M’ are located on the intersection
1 22 2 ∩ 5 26 2 =K 2, 1 22 2 ∩ 5 26 2 =K’ 2, 3 24 2 ∩ 5 26 2 =M 2, 3 24 2 ∩ 5 26 2 =M’ 2.
Other points on the intersection can be found as the intersection points of outlines: A 2, B 2, C 2,
D 2.
Sphere g min is tangent to one of intersecting surfaces and intersecting one to other surface.
Horizontal projections of the points K 1, K’ 1, M 1, M’ 1 can be determined as points of the
horizontal projection of circle 5 16 1.

78