Page 38 - 4234

parallel frontal plane of projections the right angle will be projected in the natural size on a face-to-
face plane of projections, if one party of a right angle is parallel horizontal plane of projections the
right angle will be projected in the natural size on a horizontal plane of projections.
Therefore we will take horizontal and frontal principal lines of the plane as any two stopped
straight lines.
If a line perpendicular to a plane, then the projections of the line are perpendicular to like
traces of the plane (and also to the respective projections of horizontal and frontal principal lines of
the plane).
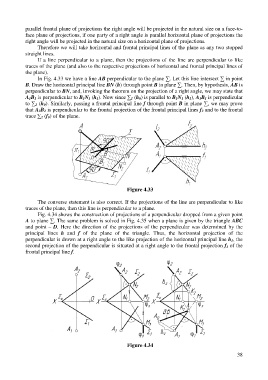
In Fig. 4.33 we have a line AB perpendicular to the plane ∑. Let this line intersect ∑ in point
B. Draw the horizontal principal line BN (h) through point B in plane ∑. Then, by hypothesis, AB is
perpendicular to BN, and, invoking the theorem on the projection of a right angle, we may state that
A 1B 1 is perpendicular to B 1N 1 (h 1). Now since ∑ 1 (h 0) is parallel to B 1N 1 (h 1), A 1B 1 is perpendicular
to ∑ 1 (h 0). Similarly, passing a frontal principal line f through point B in plane ∑, we may prove
that A 2B 2 is perpendicular to the frontal projection of the frontal principal lines f 2 and to the frontal
trace ∑ 2 (f 0) of the plane.

Figure 4.33

The converse statement is also correct. If the projections of the line are perpendicular to like
traces of the plane, then this line is perpendicular to a plane.
Fig. 4.34 shows the construction of projections of a perpendicular dropped from a given point
A to plane ∑. The same problem is solved in Fig. 4.35 when a plane is given by the triangle ABC
and point – D. Here the direction of the projections of the perpendicular was determined by the
principal lines h and f of the plane of the triangle. Thus, the horizontal projection of the
perpendicular is drown at a right angle to the like projection of the horizontal principal line h 1, the
second projection of the perpendicular is situated at a right angle to the frontal projection f 1 of the
frontal principal line f.

Figure 4.34
38