Page 39 - 4234
P. 39
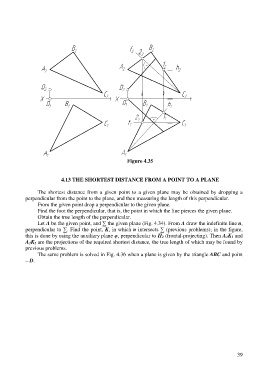
Figure 4.35
4.13 THE SHORTEST DISTANCE FROM A POINT TO A PLANE
The shortest distance from a given point to a given plane may be obtained by dropping a
perpendicular from the point to the plane, and then measuring the length of this perpendicular.
From the given point drop a perpendicular to the given plane.
Find the foot the perpendicular, that is, the point in which the line pierces the given plane.
Obtain the true length of the perpendicular.
Let A be the given point, and ∑ the given plane (Fig. 4.34). From A draw the indefinite line n,
perpendicular to ∑. Find the point, K, in which n intersects ∑ (previous problems); in the figure,
this is done by using the auxiliary plane φ, perpendicular to Π 2 (frontal-projecting). Then A 1K 1 and
A 2K 2 are the projections of the required shortest distance, the true length of which may be found by
previous problems.
The same problem is solved in Fig. 4.36 when a plane is given by the triangle ABC and point
– D.
39