Page 37 - 4234

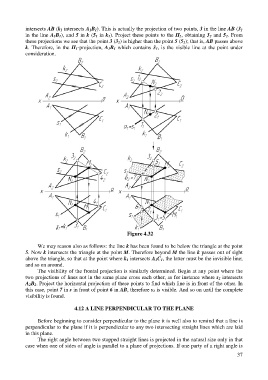
intersects AB (k 1 intersects A 1B 1). This is actually the projection of two points, 3 in the line AB (3 1
in the line A 1B 1), and 5 in k (5 1 in k 1). Project these points to the Π 2, obtaining 3 2 and 5 2. From
these projections we see that the point 3 (3 2) is higher than the point 5 (5 2); that is, AB passes above
k. Therefore, in the Π 1-projection, A 1B 1 which contains 3 1, is the visible line at the point under
consideration.

Figure 4.32

We may reason also as follows: the line k has been found to be below the triangle at the point
5. Now k intersects the triangle at the point M. Therefore beyond M the line k passes out of sight
above the triangle, so that at the point where k 1 intersects A 1C 1, the latter must be the invisible line;
and so on around.
The visibility of the frontal projection is similarly determined. Begin at any point where the
two projections of lines not in the same plane cross each other, as for instance where s 2 intersects
A 2B 2. Project the horizontal projection of these points to find which line is in front of the other. In
this case, point 7 in s in front of point 6 in AB, therefore s 2 is visible. And so on until the complete
visibility is found.

4.12 A LINE PERPENDICULAR TO THE PLANE

Before beginning to consider perpendicular to the plane it is well also to remind that a line is
perpendicular to the plane if it is perpendicular to any two intersecting straight lines which are laid
in this plane.
The right angle between two stopped straight lines is projected in the natural size only in that
case when one of sides of angle is parallel to a plane of projections. If one party of a right angle is
37