Page 36 - 4234

In the adjoining horizontal projection, determine which of the lines is more distant to the axis
x. This line is in front of the other line in the frontal projection and is visible in this projection.

Figure 4.30

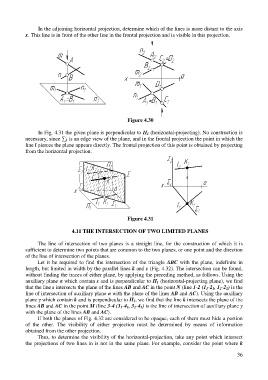
In Fig. 4.31 the given plane is perpendicular to Π 1 (horizontal-projecting). No construction is
necessary, since ∑ 1 is an edge view of the plane, and in the frontal projection the point in which the
line l pierces the plane appears directly. The frontal projection of this point is obtained by projecting
from the horizontal projection.

Figure 4.31

4.11 THE INTERSECTION OF TWO LIMITED PLANES

The line of intersection of two planes is a straight line, for the construction of which it is
sufficient to determine two points that are common to the two planes, or one point and the direction
of the line of intersection of the planes.
Let it be required to find the intersection of the triangle ABC with the plane, indefinite in
length, but limited in width by the parallel lines k and s (Fig. 4.32). The intersection can be found,
without finding the traces of either plane, by applying the preceding method, as follows. Using the
auxiliary plane α which contain s and is perpendicular to Π 1 (horizontal-projecting plane), we find
that the line s intersects the plane of the lines AB and AC in the point N (line 1-2 (1 1-2 1, 1 2-2 2) is the
line of intersection of auxiliary plane α with the plane of the lines AB and AC). Using the auxiliary
plane γ which contain k and is perpendicular to Π 1, we find that the line k intersects the plane of the
lines AB and AC in the point M (line 3-4 (3 1-4 1, 3 2-4 2) is the line of intersection of auxiliary plane γ
with the plane of the lines AB and AC).
If both the planes of Fig. 4.32 are considered to be opaque, each of them must hide a portion
of the other. The visibility of either projection must be determined by means of information
obtained from the other projection.
Thus, to determine the visibility of the horizontal-projection, take any point which intersect
the projections of two lines in is not in the same plane. For example, consider the point where k

36