Page 11 - 4234

The first and second properties are common for both of types of projection.
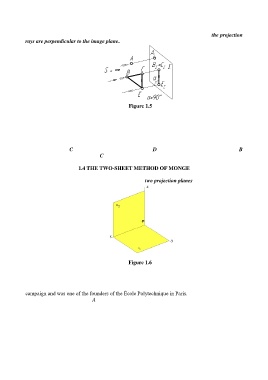
In Descriptive Geometry, however, we go one step farther and we assume that the projection
rays are perpendicular to the image plane. One simple example is shown in Figure 1.5.

Figure 1.5

Thus we obtain the orthogonal projection. This is a particular case of the parallel projection;
therefore, it inherits all the properties of the parallel projection. In addition, the assumption of
projection rays perpendicular to the image plane yields a new property as the projection of a right
angle.
But the projection on the one image plane is ambiguous identification of the spatial object.
Projection of point C coincides with projection of point D on Fig. 1.2 and projection of point B
coincides with projection of point C on Fig. 1.4 and 1.5.

1.4 THE TWO-SHEET METHOD OF MONGE

The basic idea of Descriptive Geometry is to use two projection planes (Fig.1.6).

Figure 1.6

However, the first to organize the methods for doing so in a coherent system was the French
mathematician Gaspard Monge (1746-1818). Therefore, he who is considered to be the founder of
Descriptive Geometry. Monge was one of the scientists who accompanied Bonaparte in the Egypt
campaign and was one of the founders of the Ėcole Polytechnique in Paris.
Figure 1.7 shows a point A and a system of Cartesian coordinates.

11