Page 15 - 4234

nd
down, now come below x (+Y). The  1 projections of all points behind of  2, in either the 2 ,
rd
th
3 ,6 or 7 th angles (-Y), are found above x since that part of  1 was revolved up.
st
th
th
th
The  3 projections of points in the 1 , 4 , 5 and 8 angles are on the right of z (+Y), and
nd
th
th
rd
on the left of z for the 2 3 , 6 and 7 (-Y), regardless of any revolution of planes.
As the  1 plane is revolved about x, as an axis, the  1 projections of a point moves in a
plane which is perpendicular to x, hence after the revolution the  1 projection is found on the
perpendicular to x, dropped from the  2 projection of the point. The  3 projection of a point
moves in a plane which is perpendicular to z, hence after the revolution the  3 projection is
found on the perpendicular to z, because the  3 plane is revolved about z, as an axis (Fig.2.2).

Figure 2.2

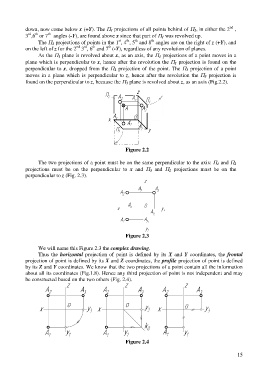
The two projections of a point must be on the same perpendicular to the axis:  1 and  2
projections must be on the perpendicular to x and  3 and  2 projections must be on the
perpendicular to z (Fig. 2.3).

Figure 2.3

We will name this Figure 2.3 the complex drawing.
Thus the horizontal projection of point is defined by its X and Y coordinates, the frontal
projection of point is defined by its X and Z coordinates, the profile projection of point is defined
by its Z and Y coordinates. We know that the two projections of a point contain all the information
about all its coordinates (Fig.1.8). Hence any third projection of point is not independent and may
be constructed based on the two others (Fig. 2.4).

Figure 2.4

15