Page 7 - 4234

CONVENTIONAL DENOTATION

Points of space are represented by capital letters: A, B, C, …
A line determined by two point A, B, is determined the line AB.
Unlimited lines of space are represented by small letters: a, b, k, …
Unlimited planes of space are represented by capital Greek letters: , …
A plane determined by three points A, B, C, is called the plane ABC.
A plane determined by two intersecting lines a and b is called plane ab.
In general, given elements are denoted by early letters of the alphabet, required elements by
late figures; given A 1, A 2, A 3, B 1, B 2, B 3, C 1, C 1, C 3, required P 1, P 2, P 3.
The projection of a point on a plane is represented by a letter for the point and a subscript for
the plane. Thus, A 1 is the projection of point A on the horizontal plane of projection Π 1. The
projection of a line is represented in the same manner. Thus, k 2 is the frontal projection or front
view of the unlimited line k. A 1B 1 is the top view or horizontal projection of the line segment AB.

In tutorial guidelines are accepted such denotation.
Points are denoted by upper-case Latin letters from A to O (except of F and H) or by Arabic
numerals.
Lines are denoted by down-case Latin letters.

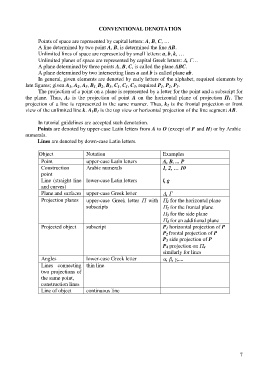
Object Notation Examples
Point upper-case Latin letters A, B, ... P
Construction Arabic numerals 1, 2, … 10
point
Line (straight line lower-case Latin letters l, g
and curves)
Plane and surfaces upper-case Greek letter , 
Projection planes upper-case Greek letter  with  1 for the horizontal plane
subscripts  2 for the frontal plane
 3 for the side plane
 4 for an additional plane
Projected object subscript P 1 horizontal projection of P
P 2 frontal projection of P
P 3 side projection of P

P 4 projection on  4
similarly for lines
Angles lower-case Greek letter , , ,....
Lines connecting thin line
two projections of
the same point,
construction lines
Line of object continuous line

7