Page 14 - 4234

2 POINT INTO SYSTEM OF 3 COORDINATE PLANES

The two conventional planes mentioned in the preceding lecture are called planes of
projection: the horizontal one, defined by the axes x and y; and the frontal one defined by the
axes x and z. But pair of axes y and z defines another plane of projection which is perpendicular
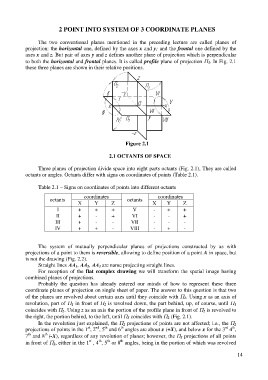
to both the horizontal and frontal planes. It is called profile plane of projection  3. In Fig. 2.1
these three planes are shown in their relative positions.

Figure 2.1

2.1 OCTANTS OF SPACE

Three planes of projection divide space into eight parts octants (Fig. 2.1), They are called
octants or angles. Octants differ with signs on coordinates of points (Table 2.1).

Table 2.1 – Signs on coordinates of points into different octants

coordinates coordinates
octants octants
Х Y Z X Y Z
І + + + V - + +
ІІ + - + VI - - +
ІІІ + - - VII - - -
ІV + + - VIII - + -

The system of mutually perpendicular planes of projections constructed by us with
projections of a point to them is reversible, allowing to define position of a point A in space, but
is not the drawing (Fig. 2.2).
Straight lines АА 1, АА 2, АА 3 are name projecting straight lines.
For reception of the flat complex drawing we will transform the spatial image having
combined planes of projections.
Probably the question has already entered our minds of how to represent these three
coordinate planes of projection on single sheet of paper. The answer to this question is that two
of the planes are revolved about certain axes until they coincide with  2. Using x as an axis of

revolution, part of  1 in front of  2 is revolved down, the part behind, up, of course, until  1
coincides with  2. Using z as an axis the portion of the profile plane in front of  2 is revolved to
the right, the portion behind, to the left, until  3 coincides with  2 (Fig. 2.1).
In the revolution just explained, the  2 projections of points are not affected; i.e., the  2
nd
rd
th
th
th
st
projections of points in the 1 , 2 , 5 and 6 angles are about x (+X), and below x for the 3 4 ,
th
th
7 and 8 (-X), regardless of any revolution of planes; however, the  1 projections of all points
th
st
th
th
in front of  2, either in the 1 , 4 , 5 or 8 angles, being in the portion of which was revolved
14