Page 9 - 4234

1 DESCRIPTIVE GEOMETRY
1.1 THE SUBJECT OF DESCRIPTIVE GEOMETRY

We live in a three-dimensional world and we often want to represent this world in a drawing,
a painting or a photography. But, drawings, paintings and photos are two-dimensional.
The aim of Descriptive Geometry is to describe the three-dimensional objects by two-
dimensional drawings so as to allow to reconstitute their original forms and their real dimensions.
So, Descriptive Geometry is a method to study 3D geometry through 2D images thus
offering insight into structure and metrical properties of spatial objects, processes and
principles.

1.2 TYPES OF PROJECTION

When representing a three-dimensional object on the two-dimensional surface of our retina, of
a camera film, of a paper sheet, or of a TV or computer screen, the number of dimensions is reduced
from three to two. The general process of reducing the number of dimensions of a given object is
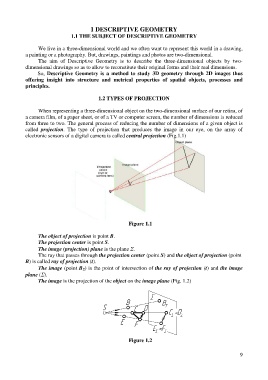
called projection. The type of projection that produces the image in our eye, on the array of
electronic sensors of a digital camera is called central projection (Fig.1.1)

Figure 1.1

The object of projection is point B.
The projection center is point S.
The image (projection) plane is the plane .
The ray that passes through the projection center (point S) and the object of projection (point
B) is called ray of projection (t).
The image (point B ) is the point of intersection of the ray of projection (t) and the image
plane ().
The image is the projection of the object on the image plane (Fig. 1.2)

Figure 1.2

9