Page 94 - 4167

viscosity is Pascal x second [Pa.s].
In common case
dv
F   S . (7.18)
dz


The quantity:   , where  is the density of the fluid, is

called kinematic viscosity.
The measurement of the dynamic viscosity of a liquid can be carried

out by special devices (apparatus) called viscosimeters.
A shear stress, denoted  (greek: tau), is defined as the
component of stress coplanar with a material cross section. Shear stress

arises from the force vector component parallel to the cross
section  F . Normal stress, on the other hand, arises from the force
S
vector component perpendicular to the material cross section on which it
acts. Therefore we can rewrite equation (7.18) in another form
dv
   . (7.19)
dz

7.6 Poiseuille's law

When a viscous fluid flows in a tube, the flow velocity is different

at different points of a cross section. The outermost layer of fluid clings
to the walls of the tube, and its velocity is zero. If the velocity is not too
great, the flow is laminar, with a velocity that is greater at the center of

the tube and decreases to zero at the walls. The flow is like that of a
number of tele-scoping tubes sliding relative to one another, the central
tube advancing most rapidly and the outer tube remaining at rest.
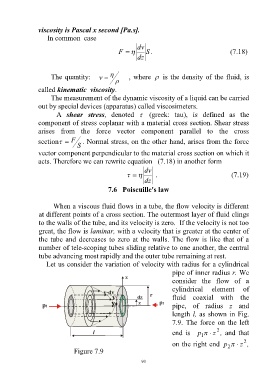
Let us consider the variation of velocity with radius for a cylindrical

pipe of inner radius r. We
consider the flow of a
cylindrical element of

fluid coaxial with the
pipe, of radius z and
length l, as shown in Fig.
7.9. The force on the left

end is p  z  2 , and that
1
on the right end p  z  2 ,
2
Figure 7.9

93