Page 96 - 4167

radius z and dS is the shaded area, equal to 2  zdz. Taking the
expression for v from eq. (7.25), we get

p  p 2 2 2
1
dV  ( r  z 2)   zdz  dt . (7.26)
l  4

The volume flowing across the entire cross section is obtained by
integrating over all elements between z = 0 and z = r.
 p (  p ) r p (  p ) r  4
2
dV  1 2  r ( 2  z 2)   zdz  dt  1 2 ..(7.27)
l  2 0 l  8
The total volume of flow per of unit time dV is called volume
dt
rate of flow and denoted by Q  dV ,therefore
dt
4
 p r 
Q  . (7.28)
l  8
This relation was first derived by Poiseuille and is called

Poiseuille's law (Jean Louis Marie Poiseuille. French physicist(1797 –
1869))
. The volume rate of flow is inversely proportional to viscosity, as
might be expected. It is proportional to the pressure gradient along the

pipe, and it varies as the fourth power of the radius.
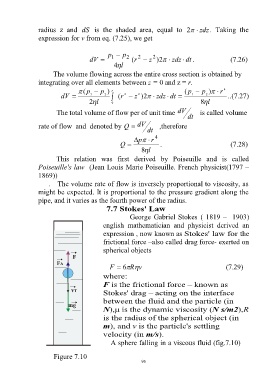
7.7 Stokes' Law
George Gabriel Stokes ( 1819 – 1903)

english mathematician and physicist derived an
expression , now known as Stokes' law for the
frictional force –also called drag force- exerted on
spherical objects

F   6 R v (7.29)

where:
F is the frictional force – known as
Stokes' drag – acting on the interface

between the fluid and the particle (in
N),μ is the dynamic viscosity (N s/m2),R
is the radius of the spherical object (in

m), and v is the particle's settling
velocity (in m/s).
A sphere falling in a viscous fluid (fig.7.10)

Figure 7.10
95