Page 73 - 4167
P. 73
6.3Work and Power of Rotational Motion
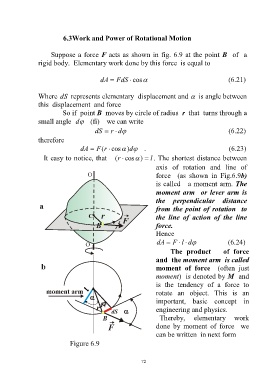
Suppose a force F acts as shown in fig. 6.9 at the point B of a
rigid body. Elementary work done by this force is equal to
dA FdS cos (6.21)
Where dS represents elementary displacement and is angle between
this displacement and force
So if point B moves by circle of radius r that turns through a
small angle d (fi) we can write
dS r d (6.22)
therefore
dA F( r cos d) . (6.23)
It easy to notice, that r cos( ) l . The shortest distance between
axis of rotation and line of
force (as shown in Fig.6.9b)
is called a moment arm. The
moment arm or lever arm is
the perpendicular distance
from the point of rotation to
the line of action of the line
force.
Hence
dA F l d (6.24)
The product of force
and the moment arm is called
moment of force (often just
moment) is denoted by M and
is the tendency of a force to
rotate an object. This is an
important, basic concept in
engineering and physics.
Thereby, elementary work
done by moment of force we
can be written in next form
Figure 6.9
72