Page 70 - 4167

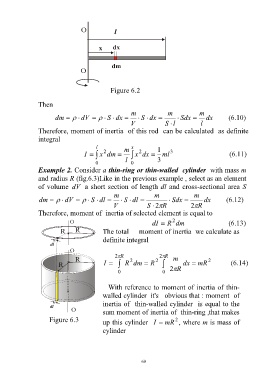
l

Figure 6.2

Then

m m m
dm    dV    S  dx   S  dx   Sdx  dx (6.10)
V S l  l

Therefore, moment of inertia of this rod can be calculated as definite
integral
l x
2 m 2 1 3
I   x dm   x dx  ml (6.11)
0 l 0 3
Example 2. Consider a thin-ring or thin-walled cylinder with mass m

and radius R (fig.6.3)Like in the previous example , select as an element
of volume dV a short section of length dl and cross-sectional area S
m m m
dm    dV    S  dl   S  dl   Sdx  dx (6.12)
V S 2  R  2 R
Therefore, moment of inertia of selected element is equal to

dI  R 2 dm (6.13)
The total moment of inertia we calculate as

definite integral

2  R 2  R
2 2 m 2
I   R dm  R  dx  mR (6.14)
0 0  2 R

With reference to moment of inertia of thin-
walled cylinder it's obvious that : moment of
inertia of thin-walled cylinder is equal to the

sum moment of inertia of thin-ring ,that makes
Figure 6.3 up this cylinder I  mR , where m is mass of
2
cylinder

69