Page 71 - 4167

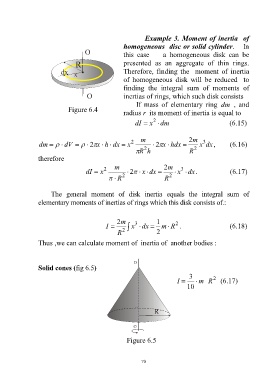
Example 3. Moment of inertia of
homogeneous disc or solid cylinder. In
this case a homogeneous disk can be

presented as an aggregate of thin rings.
Therefore, finding the moment of inertia
of homogeneous disk will be reduced to

finding the integral sum of moments of
inertias of rings, which such disk consists
If mass of elementary ring dm , and
Figure 6.4
radius r its moment of inertia is equal to
2
dI  x  dm (6.15)

m 2 m 3
2
dm    dV   2   x  h  dx  x 2   x  hdx  x dx, (6.16)
 R 2 h R 2
therefore

m 2 m 3
2
dI  x  2  x  dx   x  dx. (6.17)
  R 2 R 2

The general moment of disk inertia equals the integral sum of
elementary moments of inertias of rings which this disk consists of.:

2m 3 1 2
I   x  dx  m R . (6.18)
R 2 2
Thus ,we can calculate moment of inertia of another bodies :

Solid cones (fig 6.5)
3 2
I   m R (6.17)
10

Figure 6.5

70