Page 31 - 4167

dS
with respect to time v  So dS  r d   we get
dt
dS rd
v  
dt dt , (2.12)
d 
where   , we receive required relationship
dt
v  r 
(2.13)

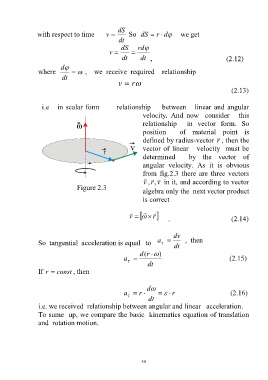
i.e in scalar form relationship between linear and angular
velocity. And now consider this

relationship in vector form. So
position of material point is

defined by radius-vector r , then the
vector of linear velocity must be

determined by the vector of
angular velocity. As it is obvious
from fig.2.3 there are three vectors
  
v r , v , in it, and according to vector
Figure 2.3
algebra only the next vector product
is correct

  
v     r
. (2.14)

dv
a  , then
So tangential acceleration is equal to 
dt
d r (  )
a  (2.15)

dt
If r  const , then

d
a  r   r   (2.16)

dt
i.e. we received relationship between angular and linear acceleration.
To sume up, we compare the basic kinematics equation of translation

and rotation motion.

30