Page 28 - 4167

2. KINEMATICS OF ROTATIONAL MOTION OF RIGID
AROUND FIXED AXIS

2.1. Main Characteristics of Rotational Motion of Rigid Body

around Fixed Axis

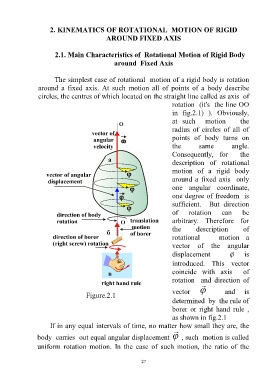
The simplest case of rotational motion of a rigid body is rotation

around a fixed axis. At such motion all of points of a body describe
circles, the centres of which located on the straight line called as axis of
rotation (it's the line OO
in fig.2.1) ). Obviously,

at such motion the
radius of circles of all of
points of body turns on

the same angle.
Consequently, for the
description of rotational

motion of a rigid body
around a fixed axis only
one angular coordinate,

one degree of freedom is
sufficient. But direction
of rotation can be
arbitrary. Therefore for

the description of
rotational motion a
vector of the angular

displacement  is
introduced. This vector
coincide with axis of

rotation and direction of

vector  and is
Figure.2.1
determined by the rule of

borer or right hand rule ,
as shown in fig.2.1

If in any equal intervals of time, no matter how small they are, the

body carries out equal angular displacement  , such motion is called
uniform rotation motion. In the case of such motion, the ratio of the

27