Page 30 - 4167


  . (2.8)
t 
Angular acceleration in SI units is measured in radians per second
2
in square (rad/s ),
In general case angular acceleration is the first derivative
of angular velocity with respect to time (compare with liner

acceleration )
d
 
dt . (2.9)
To take into consideration that angular velocity is the first

derivative of angular displacement with respect to time , angular
acceleration is the second t derivative of angular displacement
with respect to time
2
d 
  .
dt 2 (2.10)
So angular velocity is a vector, correspondingly angular

acceleration is a vector, that coincides with vector change d

 d
 
dt (2.11)
2.2. Relationships between Angular and Linear Kinematic

Values in Rotational Motion

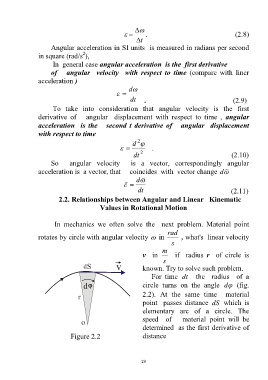
In mechanics we often solve the next problem. Material point

rad
rotates by circle with angular velocity  in , what's linear velocity
s

m
v in if radius r of circle is
s
known. Try to solve such problem.
For time dt the radius of a
circle turns on the angle d (fig.

2.2). At the same time material
point passes distance dS which is
elementary arc of a circle. The

speed of material point will be
determined as the first derivative of
Figure 2.2 distance

29