Page 87 - 4811

Application of Laplace Transform

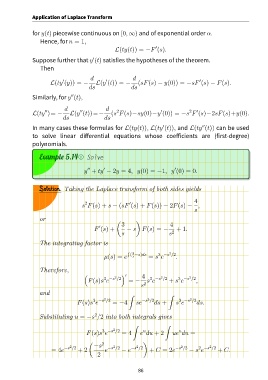
for y(t) piecewise continuous on [0, ∞) and of exponential order α.

Hence, for n = 1,
L(ty(t)) = −F (s).
′
Suppose further that y (t) satisfies the hypotheses of the theorem.
′
Then

d d
′ ′ ′
L(ty (y)) = − L(y (t)) = − (sF(s) − y(0)) = −sF (s) − F(s).
ds ds
Similarly, for y (t),
′′

d d
′′ ′′ 2 ′ 2 ′
L(ty )= − L(y (t))=− (s F(s)−sy(0)−y (0)) = −s F (s)−2sF(s)+y(0).
ds ds
In many cases these formulas for L(ty(t)), L(ty (t)), and L(ty (t)) can be used
′′
′
to solve linear differential equations whose coefficients are (first-degree)
.
polynomials.

Example 5.14, Solve

′
y + ty − 2y = 4, y(0) = −1, y (0) = 0.
′′
′
. . . . .
.
Solution. Taking the Laplace transform of both sides yields
4
2
′
s F(s) + s − (sF (s) + F(s)) − 2F(s) − ,
s
or
( )
3 4
′
F (s) + − s F(s) = − + 1.
s s 2
The integrating factor is

∫ 3 2
3 −s /2
µ(s) = e ( −s)ds = s e .
s
Therefore,
( 2 ) ′ 4 2 2
3 −s /2
3 −s /2
3 −s /2
F(s)s e = − s e + s e ,
s 2
and
∫ ∫
2
3 −s /2
3 −s /2
F(s)s e 2 = −4 se −s /2 ds + s e 2 ds.
2
Substituting u = −s /2 into both integrals gives
∫ ∫
u
3 −s /2
u
F(s)s e 2 = 4 e du + 2 ue du =
( −s 2 )
2
2
2
2
2 −s /2
= 4e −s /2 + 2 e −s /2 − e −s /2 + C = 2e −s /2 − s e 2 + C.
. . . . 2
86