Page 68 - 4811

Branch Point

F(s) → 0 (uniformly) for s on C as n → ∞. This task can be exceedingly
n
difficult and may sometimes be impossible. It is tempting for practitioners of this
technique, when F(s) has infinitely many poles, not to verify (4.13) for suitable
C . This leaves open the possibility that the resulting “inverse” function f(t) is
n
incorrect.

Branch Point

Consider the function
1
F(s) = √ ,
s
which has a branch point at s = 0. We apply the methods of the complex

inversion formula in this case.
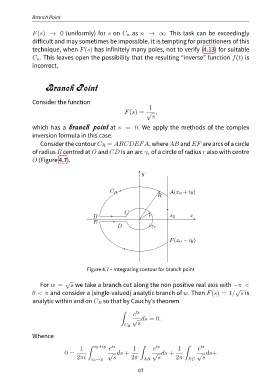
ConsiderthecontourC = ABCDEFA,whereAB andEF arearcsofacircle
R
of radius R centred at O and CD is an arc γ of a circle of radius r also with centre
r
O (Figure 4.7).

y

C R A(x 0 + iy)
R

C
B r x 0 x
E
D γ r

F(x 0 − iy)

Figure 4.7 – Integrating contour for branch point

√
For w = s we take a branch cut along the non positive real axis with −π <
√
θ < π and consider a (single-valued) analytic branch of w. Then F(s) = 1/ s is
analytic within and on C so that by Cauchy’s theorem
R
∫ ts
e
√ ds = 0.
s
C R
Whence

∫ x 0 +iy ∫ ∫
1 e ts 1 e ts 1 e ts
0 = √ ds + √ ds + √ ds+
2πi x 0 −iy s 2π AB s 2π BC s

67