Page 46 - 4811

Convolutions and Their Applications

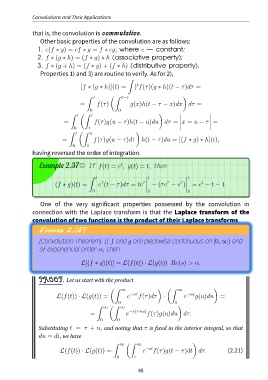
that is, the convolution is commutative.

Other basic properties of the convolution are as follows:
1. c(f ∗ g) = cf ∗ g = f ∗ cg, where c — constant;
2. f ∗ (g ∗ h) = (f ∗ g) ∗ h (associative property);
3. f ∗ (g + h) = (f ∗ g) + (f ∗ h) (distributive property).

Properties 1) and 3) are routine to verify. As for 2),
∫
t
[f ∗ (g ∗ h)](t) = ) f(τ)(g ∗ h)(t − τ)dτ =
τ t−τ
∫ (∫ )
= f(τ) g(x)h(t − τ − x)dx dτ =
0 0
t t
∫ (∫ )

= f(τ)g(u − τ)h(t − u)du dτ = x = u − τ =

0 τ
t u
∫ (∫ )
= f(τ)g(u − τ)dτ h(t − τ)du = [(f ∗ g) ∗ h](t),
0 0
.
having reversed the order of integration.
t
Example 2.37, If f(t) = e , g(t) = t, then

t
∫ t t

τ
τ
τ
t
(f ∗ g)(t) = e (t − τ)dτ = te τ − (τe − e ) = e − t − 1.

0 0 0
. . . . .
One of the very significant properties possessed by the convolution in
connection with the Laplace transform is that the Laplace transform of the
convolution of two functions is the product of their Laplace transforms.
Theorem 2.15È
(Convolution Theorem). If f and g are piecewise continuous on [0, ∞) and
of exponential order α, then

L[(f ∗ g)(t)] = L(f(t)) · L(g(t)) Re(s) > α.
. . . . . . .

PROOF. Let us start with the product

(∫ ) (∫ )
∞ ∞
L(f(t)) · L(g(t)) = e −sτ f(τ)dτ · e −su g(u)du =
0 0
∫ (∫ )
∞ ∞
= e −s(τ+u) f(τ)g(u)du dτ.
0 0
Substituting t = τ + u, and noting that τ is fixed in the interior integral, so that
du = dt, we have

∫ (∫ )
∞ ∞
L(f(t)) · L(g(t)) = e −st f(τ)g(t − τ)dt dτ. (2.21)
. . . . 0 τ

45