Page 48 - 4811

Convolutions and Their Applications
.

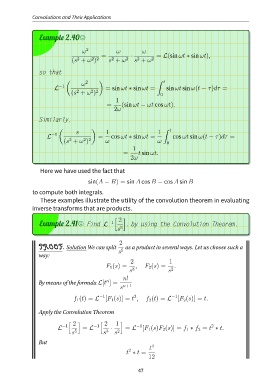
Example 2.40,

ω 2 ω ω
= · = L(sin ωt ∗ sin ωt),
2 2
2
2
2
(s + ω ) s + ω 2 s + ω 2
so that
( ) ∫ t
ω 2
−1
L = sin ωt ∗ sin ωt = sin ωt sin ω(t − τ)dτ =
2 2
2
(s + ω ) 0
1
= (sin ωt − ωt cos ωt).
2ω
Similarly,

( ) ∫ t
s 1 1
−1
L = cos ωt ∗ sin ωt = cos ωt sin ω(t − τ)dτ =
2
2 2
(s + ω ) ω ω 0
1
= t sin ωt.
2ω
. . . . .
Here we have used the fact that

sin(A − B) = sin A cos B − cos A sin B
to compute both integrals.

These examples illustrate the utility of the convolution theorem in evaluating
.
inverse transforms that are products.

[ 2 ]
−1
Example 2.41, Find L . by using the Convolution Theorem.
s 5
. . . . .
2
PROOF. Solution We can split as a product in several ways. Let us choose such a
s 5
way:
2 1
F (s) = , F (s) = .
2
1
s 3 s 2
n!
n
By means of the formula L[t ] =
s n+1
−1
−1
2
f (t) = L [F (s)] = t , f (t) = L [F (s)] = t.
1
1
2
2
Apply the Convolution Theorem
[ 2 ] [ 2 1 ]
−1 −1 −1 2
L = L · = L [F (s)F (s)] = f ∗ f = t ∗ t.
1
2
1
2
s 5 s 3 s 2
But
t 4
2
t ∗ t =
. . . . 12
47