Page 45 - 4811

Properties of Laplace Transform
.

(1)
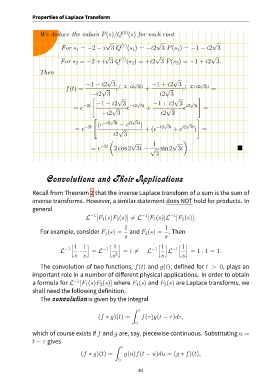
We deduce the values P(s)/Q (s) for each root
√ √ √
(1)
For s = −2 − i 3 Q (s ) = −i2 3 P(s ) = −1 − i2 3
1
1
1
√ √ √
(1)
For s = −2 + i 3 Q (s ) = +i2 3 P(s ) = −1 + i2 3.
2
2
2
Then
√ √
−1 − i2 3 √ −1 + i2 3 √
f(t) = √ e (−2−i2 3)t + √ e (−2+i2 3)t =
−i2 3 i2 3
[ √ √ ]
−1 − i2 3 √ −1 + i2 3 √
= e −2t √ e −i2 3t + √ e i2 3t =
−i2 3 i2 3
[ √ √ ]
(e −i2 3t − e i2 3t ) √ √
= e −2t √ + (e −i2 3t + e i2 3t ) =
i2 3

( )
√ 1 √
= e −2t 2 cos 2 3t − √ sin 2 3t .
3
. . . .

Convolutions and Their Applications

Recall from Theorem 2 that the inverse Laplace transform of a sum is the sum of
inverse transforms. However, a similar statement does NOT hold for products. In
general
−1
−1
−1
L [F (s)F (s)] ̸= L [F (s)]L [F (s)].
2
2
1
1
1 1
For example, consider F (s) = and F (s) = . Then
2
1
s s
1
1
[ 1 1 ] [ 1 ] [ ] [ ]
−1 −1 −1 −1
L · = L = t ̸= L L = 1 · 1 = 1.
s s s 2 s s
The convolution of two functions, f(t) and g(t), defined for t > 0, plays an
important role in a number of different physical applications. In order to obtain
−1
a formula for L [F (s)F (s)] where F (s) and F (s) are Laplace transforms, we
1
2
2
1
shall need the following definition.
The convolution is given by the integral
t
∫
(f ∗ g)(t) = f(τ)g(t − τ)dτ,
0
which of course exists if f and g are, say, piecewise continuous. Substituting u =
t − τ gives
∫
t
(f ∗ g)(t) = g(u)f(t − u)du = (g ∗ f)(t),
0

44