Page 111 - 4660

Base concepts

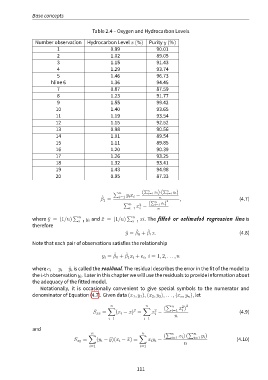
Table 2.4 – Oxygen and Hydrocarbon Levels

Number observation Hydrocarbon Level x (%) Purity y (%)
1 0.99 90.01
2 1.02 89.05
3 1.15 91.43
4 1.29 93.74
5 1.46 96.73
hline 6 1.36 94.45
7 0.87 87.59
8 1.23 91.77
9 1.55 99.42
10 1.40 93.65
11 1.19 93.54
12 1.15 92.52
13 0.98 90.56
14 1.01 89.54
15 1.11 89.85
16 1.20 90.39
17 1.26 93.25
18 1.32 93.41
19 1.43 94.98
20 0.95 87.33

∑ n ( ∑ n x i)·( ∑ n y i)
i=1
i=1
ˆ
β 1 = i=1 y i x i − n 2 , (4.7)
∑ ( ∑ n x i)
n 2 i=1
x −
i=1 i n
∑ n ∑ n
where ¯y = (1/n) y i and ¯x = (1/n) xi. The fitted or estimated regression line is
i=1 i=1
therefore
ˆ
ˆ
ˆ y = β 0 + β 1 x. (4.8)
Note that each pair of observations satisfies the relationship
ˆ
ˆ
y i = β 0 + β 1 x i + e i , i = 1, 2, . . . , n
where e i = y i − ˆy i is called the residual. The residual describes the error in the fit of the model to
the i-th observation y i . Later in this chapter we will use the residuals to provide information about
the adequacy of the fitted model.
Notationally, it is occasionally convenient to give special symbols to the numerator and
denominator of Equation (4.7). Given data (x 1 , y 1 ), (x 2 , y 2 ), . . . , (x n , y n ), let

n n ∑ n 2 2
∑ ∑ ( x )
2
2
S xx = (x i − ¯x) = x − i=1 i (4.9)
i
i=1 i=1 n
and
n n ∑ n ∑ n
∑ ∑ ( x i ) ( y i )
S xy = (y i − ¯y)(x i − ¯x) = x i y i − i=1 i=1 (4.10)
n
i=1 i=1

111