Page 89 - 4624

b

a c
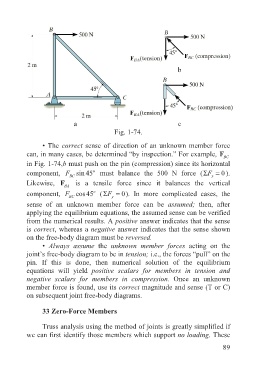
Fig. 1-74.

• The correct sense of direction of an unknown member force
can, in many cases, be determined “by inspection.” For example, F BC
in Fig. 1-74,b must push on the pin (compression) since its horizontal
Σ
component, F BC sin45 must balance the 500 N force ( F = ).
o
0
x
Likewise, F is a tensile force since it balances the vertical
BA
o
component, F BC cos45 ( F = 0). In more complicated cases, the
Σ
y
sense of an unknown member force can be assumed; then, after
applying the equilibrium equations, the assumed sense can be verified
from the numerical results. A positive answer indicates that the sense
is correct, whereas a negative answer indicates that the sense shown
on the free-body diagram must be reversed.
• Always assume the unknown member forces acting on the
joint’s free-body diagram to be in tension; i.e., the forces “pull” on the
pin. If this is done, then numerical solution of the equilibrium
equations will yield positive scalars for members in tension and
negative scalars for members in compression. Once an unknown
member force is found, use its correct magnitude and sense (T or C)
on subsequent joint free-body diagrams.

33 Zero-Force Members

Truss analysis using the method of joints is greatly simplified if
we can first identify those members which support no loading. These
89