Page 8 - 4560

spatial bending, when external forces act in different planes
(planes of bending moments at various cross-sections are oriented
differently) and elastic beam line is a spatial curve.
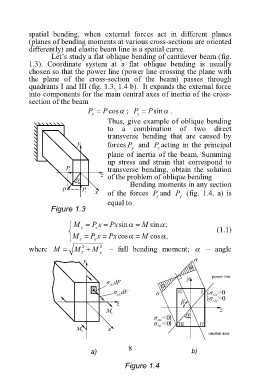
Let’s study a flat oblique bending of cantilever beam (fig.
1.3). Coordinate system at a flat oblique bending is usually
chosen so that the power line (power line crossing the plane with
the plane of the cross-section of the beam) passes through
quadrants I and III (fig. 1.3, 1.4 b). It expands the external force
into components for the main central axes of inertia of the cross-
section of the beam
P  P cos ; P  P sin .
y z
Thus, give example of oblique bending
to a combination of two direct
transverse bending that are caused by
forces P and P acting in the principal
y z
plane of inertia of the beam. Summing
up stress and strain that correspond to
transverse bending, obtain the solution
of the problem of oblique bending
Bending moments in any section
of the forces P and P (fig. 1.4, a) is
z y
equal to
Figure 1.3

 M  P x  Px sin  M sin ;
z
y
 (1.1)
 M  P x  Px cos  M cos ,
y
z
2
2
where M  M  M – full bending moment;  – angle
z y

8
a) b)

Figure 1.4