Page 12 - 4560

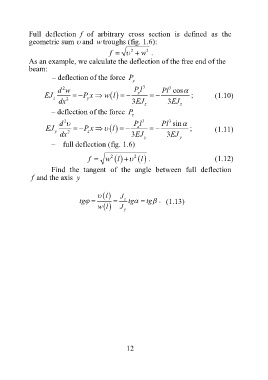
Full deflection f of arbitrary cross section is defined as the
geometric sum  and w troughs (fig. 1.6):
2
2
f    w .
As an example, we calculate the deflection of the free end of the
beam:
– deflection of the force P
y
2
d w P l 3 Pl 3 cos
y
EJ   P x  w   l     ; (1.10)
z 2 y
dx 3EJ 3EJ
z z
– deflection of the force P
z
2
d  Pl 3 Pl 3 sin
EJ   P x     l   z   ; (1.11)
y 2 z
dx 3EJ 3EJ
y y
– full deflection (fig. 1.6)

f  w 2   l   2   l . (1.12)
Find the tangent of the angle between full deflection
f and the axis y

   l J
tg   z tg  tg . (1.13)
w   l J
y

12